Action schemas | Advanced Mathematical Thinking | Algebra | Ambiguity in thinking | APOS Theory | Attitudes to mathematics | Axioms for Infinitesimal Calculus

BAPOS Theory | Bifurcation in thinking | Blancmange function

Calculus (& Computers) | Catastrophe theory | Chaos theory | Cognitive Development in Mathematics | Cognitive Units | Cognitive Roots | Cognitive Kit-bags | Cognitive Collages | Cognitive obstacles | Compression of concepts | Concept Image and Concept Definition | Concept maps and schematic diagrams | Conflicts in thinking | Continuity

Differential equations | Divergence in cognitive growth | Duality in thinking

Elementary mathematical thinking | Embodied objects

Facets and Layers of concepts | Formal Thinking (see Natural Thinking)

Gender | Generic limits | Generic organizers | Generic tangents | Generic thinking | Giving and extracting meaning | Grounded theory

Implicit functions | Inconsistencies in thinking | Infinity (cardinal) (ordinal) (measuring) (non-standard) | infinitesimals | instrumental and relational understanding | intuition and rigour

Knowledge Structure

Layers of a concept | Limit concept (see also generic limit) | local linearity | local straightness | long-term learning schema |

Met-befores | Making and having concepts | measuring infinity | modes of mathematical thinking

Natural and formal thinking | natural and formal infinity | neurophysiology | number development

Obstacles (see cognitive obstacles) | operable definitions | organizing principle

PAR Theory | Problem Solving | Procedural and conceptual learning | Procedure-process-procept | Procept (operational) (potential) (potentially infinite) (structural) (implicit) | Process-object encapsulation | Proof | Pseudo-rationals | Pseudo-irrationals

Set-before | Schema | Schematic Diagrams (see Concept Maps)

Thinkable Concept | Thought experiment (see also Proof) | Three worlds of mathematical thinking

Van Hiele development | Versatile thinking | Visualization | Visual organizer

Worlds of Mathematical Thinking

Action schema (See also Schema)
An action-schema in the sense of Piaget is a connected sequence of cognitive actions, such as 'see-grasp-suck'. An action-schema in mathematical thinking is a regular succession of activities developed by the individual, which occurs sequentially in time.

Advanced Mathematical Thinking
Advanced Mathematical thinking, as a term used in my own publications, refers to the formulation developed by Gontran Eryvnck for the Advanced Mathematical Thinking group of PME, in the book of the same name, edited by David Tall and published by Kluwer.

As a first approximation, advanced mathematical thinking focuses on the whole cycle of creation of formal mathematics, including the creation of new mathematical ideas and the formulation of these ideas in an axiomatic mathematical theory. Elementary mathematical thinking [involving calculations in arithmetic, manipulations in algebra and description and deduction in geometry becomes advanced mathematical thinking when the concept images in the cognitive structure are reformulated as concept definitions and used to construct formal concepts that are part of a systematic body of shared mathematical knowledge. (Tall, 1995).

Algebra
Algebra is a major theme in my research. For further details, click here.

Ambiguity in thinking
Mathematics is usually considered the most logical and precise of human activities. However, in studying how we think about mathematics, we find that it is useful to be able to switch from one aspect to another at will. For instance 2+3 evokes both a process of addition and a concept of sum. I claim that ambiguity is an essential feature of mathematical thinking (see Gray & Tall, 1994).

APOS Theory
APOS Theory is a theory of cognitive development, developed by Ed Dubinsky and his colleagues, based on Piaget’s notion of reflective abstraction. Actions are performed on existing (physical or mental) objects, transformed into Processes which can be conceived as a whole without carrying out individual steps, encapsulated into mental Objects that are built into a wider Schema of ideas. Schemas can themselves also be encapsulated as Objects.

My main difficulties with this theory is that it fails to focus on the nature of the initial objects acted upon (wherein the actions cause observable transformations whose effect can be the focus of attention and give rise to a mental concept). APOS begins the cycle with actions that are 'external to the user' and therefore need not make initial sense in themselves; it does not focus on the existence of intuitive embodied concepts that may be a source of initial meaning and distrusts the use of visual and embodied reasoning prior to transformation into formal thinking.

Attitudes to mathematics
I do not study attitudes to mathematics as much as it deserves. However, the research of Yudariah Yusof is a major theme, here classified under problem-solving.

Axioms for Infinitesimal Calculus
In my article of the same name, I introduce a set of axioms for infinitesimal calculus which are essentially a set-theoretic version of the logical axioms introduced by Kiesler. See my article for details.

BAPOS Theory
A variant of the APOS theory of Ed Dubinsky's theory of Action, Process, Object, Schema that takes account of the initial objects on which the actions operate. BAPOS theory begins with Base Objects on which the individual performs Actions, coordinated into Processes, represented by symbols having meaning as mental Objects, within a wider Schema. The essence of BAPOS theory is that the mental schema embraces both perceived manipulations on the base objects and the corresponding symbolic ideas. An example is the putting together of two and three (base) objects and the counting of the result to get five; this is formulated in the mathematical symbolism for 2+3 = 5.

The precise mechanism by which the encapsulation of process as object occurs was proposed by Anna Poynter in her PhD thesis. Anna found that the actions on the base objects and the change in focus to process occurs by focussing on the effect of the operations. Different actions can have the same effect and by focusing on the effect rather than the details of the action, the student can comprehend the notion of process and, more important, by using symbols to represent the effect, the properties of the effects give the properties of the symbols and hence help to turn it into an Object.

Bifurcation in thinking
This is is another term for the proceptual divide.

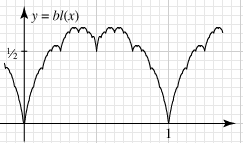
Blancmange function The blancmange function is an everywhere continuous, nowhere differentiable function that is easy to draw and to visualize. For more information, download this article in PDF.

Calculus (& Computers)
This is a major theme of my research.

Catastrophe theory
A branch of mathematics that studies systems where a continuous change in a control parameter causes a discontinuous change in the system. I used catastrophe theory to model cognitive change in my early papers. Conflicts and Catastrophes in the learning of mathematics (1976), Cognitive Conflict and the learning of mathematics (PME, 1977)

Chaos theory
A branch of mathematics that studies long-term change which is sensitive to initial conditions, so that small initial changes cause great differences long-term. The cognitive development of students learning chaos theory was interpreted using BAPOS theory by my student Soo Duck Chae.

Cognitive Development in Mathematics
This is a major theme of my research.

Cognitive Unit
A cognitive unit consists of a cognitive item that can be held in the focus of attention of an individual at one time, together with other ideas that can be immediately linked to it. (Tall & Barnard, in progress, Barnard & Tall, 1997, Crowley & Tall, 1999).

Cognitive Root
Definition: A cognitive root is a concept that:
(i) is a meaningful cognitive unit of core knowledge for the student at the beginning of the learning sequence,
(ii) allows initial development through a strategy of cognitive expansion rather than significant cognitive reconstruction,
(iii) contains the possibility of long-term meaning in later developments,
(iv) is robust enough to remain useful as more sophisticated understanding develops.
(Tall, McGowen, DeMarois, 2000, based on Tall, 1989)

Cognitive obstacle
The fundamental idea was formulated by Gaston Bachelard in La formation de l’esprit scientifique,(1938):
“We encounter new knowledge which contradicts previous knowledge, and in doing so must destroy ill-formed previous ideas.”
I use the term to refer to a way of thinking in a given context (eg 'multiplication makes bigger' dealing with whole numbers) which does not hold in a new context (multiplication of fractions). The French School (particularly Brousseau) identify a range of different kinds of obstacles, including '... psychological obstacles which occur as a result of the personal development of the student, didactical obstacles which occur because of the nature of the teaching and the teacher, and epistemological obstacles which occur because of the nature of the mathematical concepts themselves' (Cornu, Advanced Mathematical Thinking, ed Tall). The most difficult obstacles seem to be those that are endemic in the cognitive development of the subject that are resistant to a range of didactical approaches. For instance, the notion of limit is seen by many learners as an ongoing process which never attains its 'final value', for instance, '0.9 recurring' is 'just less than one'.

Compression of concepts
The term compression has been introduced only recently into mathematics education. I was first attracted by the following quote:

Mathematics is amazingly compressible: you may struggle a long time, step by step, to work through some process or idea from several approaches. But once you really understand it and have the mental perspective to see it as a whole, there is often a tremendous mental compression. You can file it away, recall it quickly and completely when you need it, and use it as just one step in some other mental process. The insight that goes with this compression is one of the real joys of mathematics. (Thurston, W. P.: 1990. Mathematical Education, Notices of the American Mathematical Society, 37 7, 844-850. qote on p.847.)

The idea of compression also has a sound neurophysiological basis:

As a task to be learned is practiced, its performance becomes more and more automatic; as this occurs, it fades from consciousness, the number of brain regions involved in the task becomes smaller. (Edelman, G. M. & Tononi, G. (2000). Consciousness: How Matter Becomes Imagination. New York: Basic Books, p.51)

It also relates to the 'compression' of ideas in the focus of attention. See Barnard & Tall (submitted).

Computers in School and College Mathematics
This is a major theme in my research.

Concept Image and Concept Definition

The following is taken directly from ‘Concept Image and Concept Definition in Mathematics’ (Tall & Vinner, 1981).

The term concept image [is used] to describe the total cognitive structure that is associated with the concept, which includes all the mental pictures and associated properties and processes. It is built up over the years through experiences of all kinds, changing as the individual meets new stimuli and matures. ... We shall call the portion of the concept image which is activated at a particular time the evoked concept image. At different times, seemingly conflicting images may be evoked. ... We shall regard the concept definition to be a form of words used to specify that concept. It may be learnt by an individual in a rote fashion or more meaningfully learnt and related to a greater or lesser degree to the concept as a whole. It may also be a personal reconstruction by the student of a definition.

(Note that this definition differs from that used by Shlomo Vinner in other papers, where he separates concept image and definition as to distinct 'cells'. My view is that both concept image and definition become part of the individual's cognitive structure and therefore the (personal) concept definition is part of the concept image. There should be no difficulty in using either of these slightly different forms together.)

Concept Maps and Schematic Diagrams

Concept maps have various shades of meaning in the literature. In her PhD thesis, Mercedes McGowen defined:

A concept map is a diagram representing the conceptual structure of a subject discipline as a graph in which nodes represent concepts and connections represent cognitive links between them. Given a sequence of concept maps, a schematic diagram for the second and successive maps is an outline diagram for each distinguishing:

• items from the previous concept map remaining in the same position,
• items from the previous concept map moved somewhere else,
• items in the current map not in the previous map.

Analysing successive schematic diagrams in a study of college students studying algebra revealed a bifurcation in which successful students build on old knowledge by extending their previous concept maps, but less successful students seem to reconstruct their concept maps anew on each successive occasion.

Conflicts in thinking
A conflict (or inconsistency) in thinking occurs when there are two (or more) distinct ways of interpreting data that are not coherent. This occurs far more often in mathematical development than is apparent. It occurs in particular when experience in one context leads to incidental properties that do not carry over to other concepts. For instance, in counting whole numbers, after each number there is a 'next' number and there are no numbers between one number and the next. In fractions, there is no 'next' number and between any two fractional numbers there is an infinite number of fractions.

Continuity
is a concept that has different meanings in different contexts. In everyday language, 'continuity' refers to a smooth variation of some variable quantity. In formal mathematics, continuity is given a formal epsilon-delta definition. These two conceptual structures are quite different. For instance, a continuous graph in everyday terms is a graph that can be drawn without taking the pencil off the paper. The function f defined on the integers by f(x)=x is continuous in the formal epsilon-delta sense, but it is not continuous in the everyday sense because the graph consists of discrete points (1,1), (2,2) etc. My own personal contribution is a visual graphic definition of continuity: A continuous function defined on a domain D (of real numbers) is a function that can be 'pulled flat', ie, the picture seen through a square window of fixed size on a computer pulls out flat when focusing on any point x and maintaining a fixed vertical ratio while expanding the horizontal scale. Essentially, give a central point a, with a pixel height ±epsilon, then there is an interval delta so that pulling the range from a-delta to a+delta to fill the whole horizontal range in the computer window reveals the graph in f(x)±epsilon. In Tall 2003a, I show how this embodied idea of 'pulling flat' is a cognitive root for the idea of continuity.

Differential equations
I have a significant difficulty with what I regard as the generally accepted notion of differential equation as an equation in the form:

dy/dx=f(x,y)

where 'y is a function of x' and dy/dx is the derivative of y with respect to x. This is a derivative equation, not a differential equation.

For me, a functional relation between two variables in x, y in embodied terms is a subset of the plane which can be traced by moving a finger over the points (x,y) in the subset. For instance, x²+y²=constant is a functional relationship. In formal terms, a functional relationship is a set of points that can be (locally) parametrised in the form x=x(t), y=y(t) where one can think of this as drawing the finger along a curve in time t. If the parametrisation is differentiable, an increment in t of size dt gives a tangential increment dx=x'(t) dt and dy=y'(t) dt. The vector (dx, dy) is then the direction of the tangent vector to the curve in the functional relationship. The differential equation therefore gives the direction of the tangent vector along a (parametrised) functional relationship.

A discussion of some of these ideas can be found in the early article Tall 1986a entitled lies, damn lies and differential equations. Other papers include Tall 1992b which looks at the essential meaning of differential and more recently in Tall 2003a which suggests and embodied approach ton the calculus.

It is my firm contention that the idea that a differential equation gives the direction of a locally straight curve is a cognitive root for the notion of differential equation in general. This idea easily extends to first order differential equations in several variables dx/dt=F(x,y,t), dy/dt=G(x,y,t)... to higher order differential equations d²x/dt² = F(t,x,dx/dt) and more generally to systems of ordinary differential equations.

Divergence in cognitive growth
The divergence between those who use interpret processes only as procedures and therefore make mathematics harder for themselves, and those that see them as flexible procepts (Gray & Tall, 1991).

Duality in thinking
In the use of symbols as procepts, duality refers to the way in which a symbol has a dual role as process or concept. For example, 3+4 is the process of addition or the concept of sum.

Elementary mathematical thinking
This is a term used by the Advanced Mathematical Thinking Group of PME to distinguish between their interests in post-16 mathematics where formal proof begins to play a fundamental role and earlier 'elementary mathematical thinking' including arithmetic, school algebra and geometry.

Embodied objects
The notion of embodied object 'begins with sensory perception and is refined in mental thought through the use of language to give increasingly refined precision and hirearchies of meaning.' (Gray and Tall, 2001i). Essentially an embodied object is one that is conceptualised sensorily within the mind. This is different from a procept which arises through symbolizing the encapsulation of a process.

Formal Thinking (see Natural Thinking)

Facets and Layers of concepts
These terms were introduced by Phil DeMarois in his PhD thesis. A facet is a particular representation of a concept (eg a function has not only algebraic, numeric and geometric facets, but also verbal, written, kinaesthetic and notational facets), a layer is a level of compression in proce-object encapsulation (eg pre-procedure, procedure, process, object, procept). See DeMarois and Tall (1993e).

Gender
Only one of my papers considers the question of gender, giving empirical data from the thesis of Norman Blackett (Blackett & Tall 1991). In this study, females using computers cooperatively to study the geometric ideas of trigonometry improved significantly better than males, reversing the advantage that the males had at the beginning of the study.

Generic Limit
A generic limit is a limiting object conceived as having the same properties as the objects in the limiting process. For example, the generic limit of 0.9, 0.99, 0.999 is 0.999... which is less than 1. (David Tall, PhD thesis 1986.)

Generic Organizer
a generic organizer is an environment (or microworld) which enables the learner to manipulate examples and (if possible) non-examples of a specific mathematical concept or a related system of concepts (David Tall, PhD thesis 1986, discussed in Tall 1998e). This is formulated as a complementary construction of an advance organizer (Ausubel) who saw the latter as a higher level structure to organize learning whereas a generic organizer builds up from examples.

Generic Tangent
'An imagined line touching the graph at only one point (even where this is inappropriate)' (Tall 1987)

Generic thinking
Thinking of specific instances of a concept as representing the general idea itself. For example, giving the example 2+3=3+2 to represent the general concept of commutativity of addition.

Giving and extracting meaning
Developing a formal theory from a concept definition can be done in at least two ways: giving meaning to the definition from one's concept image, reconstructing it as necessary on the way, or extracting meaning from the definition by deduction. This analysis was developed by Marcia Pinto in her thesis (1998). See Pinto and Tall (1999).

Grounded theory
A method formulated by Strauss and Corbin (Basics of qualitative research, Sage, 1990) that categorises empirically collected data to build a general theory to fit the data. (Used in several research projects, particularly in the thesis of Marcia Pinto). Having observed several studies using grounded theory, I find it essentially unsatisfying. Without a sense of what one is looking for, simply collecting a lot of data then looking for links and themes can be not only long and exhausting, it can lead to a theory that puts together links in an idiosyncratic way. My own belief is that it is better to begin with an exploratory study to seek out possible connections, then to formulate specific conjectures to seek data that supports, refines or conflicts with the conjecture.

Implicit function
Loosely described as a relationship between two or more variables that may or may not be represented as one variable being a function of the other. The topic is a bete-noir of the calculus curriculum. My own approach occurred through realising that an implicit function such as x²+y²=1 is not an arbitrary relation between x and y. It is a set of points in the plane that I can trace my finger over. Hence it should be approached as a set that can be parametrised as x=x(t), y=y(t) (at least locally). This is a more appropriate way to deal with differentials in the calculus. See Tall 1992b.

Inconsistencies in thinking
see cognitive conflict

Infinity (Natural) (Formal) (Cardinal) (Ordinal) (Measuring) (Non-standard)
Infinitesimals
Instrumental and relational understanding
Intuition and rigour
Limit (see also generic limit)
Local linearity
A function f is said to be locally linear at a point x in its domain if the graph has a linear approximation at that point. This seeks a symbolic representation of a linear function approximating to the curve using a limiting procedure to calculate the best linear fit perhaps with a formal epsilon-delta construction. As the fixed point is varied, the symbolic representation of the locally linear function gives the global derivative function.
Local straightness
A function f is said to be locally straight at a point x in its domain if it looks like a straight line under high magnification. Technically, one needs to know that this straightness is eventually stable as the magnification increases. Local straightness is an embodied concept. It involves looking along the curve and seeing the changing slope as a function in its own right, calculating the slope numerically to get a good-enough approximation at a point, or symbolising the slope precisely as the derivative over the whole domain. A locally straight approach to calculus uses a computer to dynamically explore the changing slope of the graph of a function, conceptualising the resulting slope as a slope function, then approximating the slope at a point numerically, or seeking the perfect symbolic expression for the stabilised slope graph.
Long-term learning schema
Making and having concepts
Measuring Infinity
Met-before
A working definition of a ‘met-before’ is ‘a structure we have in our brains now as a result of experiences we have met before.’A met-before can be supportive in a new situation, or it can be problematic. For instance, the met-before ‘2+2 is 4’ is supportive not only in its original context of counting objects or fingers, but throughout the development of number systems to real numbers and even complex numbers. The met-before ‘take away leaves less’ works for whole numbers, even for (positive) fractions, but it is problematic with negative numbers, where taking away a negative number gives more. It is also problematic in the theory of infinite cardinal numbers where two sets are defined to have the same cardinal number (allowing us to say they are the same size) if their elements can be placed in one-one correspondence. The set of natural numbers and its subsets of even numbers and odd numbers, all have the same cardinal number using the mapping from n to 2n to . Taking away the even numbers from the natural numbers leaves the odd numbers which are the same size as the full set.
A met-before (take away leaves less) can be supportive in some contexts (whole numbers, lengths, areas) yet problematic in others (negative numbers, infinite cardinal numbers).

Modes of Mathematical Thinking:
1. (Conceptual) Embodied
Conceptual embodiment builds on human perceptions and actions developing mental images that are verbalized in increasingly sophisticated ways and become perfect mental entities in our imagination.

2. (Operational) Symbolic
Operational symbolism grows out of physical actions into mathematical procedures. While some learners may remain at a procedural level, others may conceive the symbols flexibly as operations to perform and also to be operated on through calculation and manipulation.
(The operational symbolic was earlier entitled 'proceptual symbolic'. It is now conceptualized as including both procedural and proceptual operational thinking.)

3. (Axiomatic) Formal
Axiomatic formalism builds formal knowledge in axiomatic systems specified by set-theoretic definition, whose properties are deduced by mathematical proof.

Natural and Formal Thinking
Neurophysiology
Number development
Obstacles (see cognitive obstacles)
Operable definitions
Organizing principle
PAR Theory
Problem Solving
Procedural and conceptual knowledge
Procept
A procept is, essentially, a symbol that can be used dually to evoke either a process or the output of that process. Gray & Tall, 1994a gave the following definitions:

An elementary procept is the amalgam of three components: a process which produces a mathematical object and a symbol which is used to represent either process or object.

A procept consist of a collection of elementary procepts which have the same object.

For example, 3+4 is an elementary procept which can also be symbolised as the number 7. The procept 7 here consists of a collection of elementary procepts such as 4+3, 9-2 etc. It can begin as a single elementary procept and increase in sophistication as the individual matures.

Procepts can be of different kinds requiring different kinds of cognitive construction and causing different kinds of cognitive problem. These include:
...operational
an operational procept is a procept that has a built-in produre to give a result. For instance, 3+4 has a built-in procedure (counting) to give the result 7.
...potential
a potential procept is one that only has a potential process of evaluation, in particular and algebraic expression 2+3x, whose 'result' is only known when x is known.
...potentially infinite
a potentially infinite procept is a procept given by a limiting process. Here there may not even be a finite procedure to calculate the result.
...implicit
an implicit procept is a procept such as the powers x^1/2 or x^-1 whose value is implicit in the context (in this case given by the power law). Another name for a 'structural procept'.
...structural
a structural procept is one in which there is not necessarily a direct procedure to give the result of the process involved. It must instead be constructed from the given structure in the context. Another name for 'implicit procept'.
For more detail, see Symbols and the bifurcation between procedural and conceptual thinking (Tall, Gray, et al 2001)

Proceptual thinking
The ability to switch between symbols as process to do and concept to think about. The symbols in this case must be procepts which are capable of evoking either process or concept.

We characterize proceptual thinking as the ability to manipulate the symbolism flexibly as process or concept, freely interchanging different symbolisms for the same object. It is proceptual thinking that gives great power through the flexible, ambiguous use of symbolism that represents the duality of process and concept using the same notation. (Gray & Tall, 1994).

Proceptual divide
This is the widening chasm of operation in arithmetic (and later in algebra etc) between those who develop increasingly flexible thinking and those who remain at best with naive procedures.

It is our contention that whilst more able younger children evoke proceptual thinking to use the few combinations already known to establish more, less able children remain concerned with the procedures of counting and apply their efforts to developing competence with them. Procedural thinking in the context of developing competency with the number combinations can give guaranteed success and efficiency within a limited range of problems. But this efficiency with small numbers is unlikely to lead to success with more complex problems as the children grows older. Their persistence in emphasising procedures leads many children inexorably into a cul-de-sac from which there is little hope of future development.
This lack of a developing proceptual structure becomes a major tragedy for the less able which we call the proceptual divide. We believe it to be a major contributory factor to widespread failure in mathematics. (Gray & Tall, 1994).

Process-Object Encapsulation
This refers to the manner in which a process (such as counting) becomes encapsulated as a concept (number). The precise process by which this shift occurs is not absolutely clear, but I believe that the use of a symbol representing either is pivotal, allowing the individual to switch between process to carry out a manipulation or transformation of some kind and mental object, thinking of the symbol as a manipulable entity. Anna Poynter has shown that focusing on the effect of the process is pivotal in switching from process to object. For instance, in counting, there may be many ways in which the elements of a given set may be counted. However, they all have the same effect, ending on the same number which is the number of elements in the set.

Proof
Schemas
Thought experiment (see also proof)
Van Hiele development
Versatile thinking
Visualization
Visual organizer

Worlds of Mathematical Thinking
The embodied, symbolic and formal modes of mathematical thinking involve quite different 'warrants for truth' to satisfy the individual that the ideas involved are sound. The embodied world relies on perception. Something is true if it is 'seen' to be true. The proceptual world relies on calculation (or symbol manipulation). An arithmetic or algebraic statement is true through symbolic checking. The formal world relies on proof based on concept definitions.

The three worlds of mathematical thinking are:

Conceptual embodiment builds on human perceptions and actions developing mental images that are verbalized in increasingly sophisticated ways and become perfect mental entities in our imagination.

Operational symbolism grows out of physical actions into mathematical procedures. While some learners may remain at a procedural level, others may conceive the symbols flexibly as operations to perform and also to be operated on through calculation and manipulation.

Axiomatic formalism builds formal knowledge in axiomatic systems specified by set-theoretic definition, whose properties are deduced by mathematical proof.

THREE WORLDS OF MATHEMATICS

As I worked on various aspects of mathematics through the years I began to realise that there were three very different developments that occur in mathematics based on our perceptions of objects, actions on objects which are represented by symbols that can be manipulated as mental objects, and on the properties of physical and mental objects that we reflect upon and use as a basis for deduction. This led me to categorise mathematical thinking into three different worlds: a conceptual-embodied world of objects perceived and conceived, a proceptual-symbolic world of symbols (as process and concept) that arise from symbolising actions, subsequently renamed the operational-symbolic world to include both procedural and proceptual, and an axiomatic-formal world that arises from properties that are defined and concepts that are constructed through formal proof. I found that each world develops quite differently, with different cognitive sequences of development, different uses of language and different forms of proof. For more information on advanced mathematical thinking, click here.