|
The final program in this section
considers heterogeneity in the exposed and infectious cases,
effectively using the time-since infection to descriminate differences
in behaviour. This formulism means that we have far greater control
over the distribution of times that an individual remains in the
exposed and infectious classes. The basic equations for a disease with m exposed classes and n-m infectious classes is:
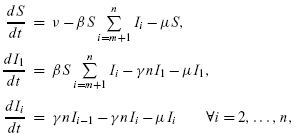
When n=2
and m=1 we return to the
standard SEIR model, with exponentially distributed periods; however as
n and m become large the distributions
become closer to fixed times.
| β |
is the transmission
rate and incorporates the encounter rate between susceptible and
infectious individuals together with the probability of transmission. |
| γ |
is
called the removal
or recovery rate, though often we are more interested in its reciprocal
(1/γ) which determines the average infected period. Note that movement
between classes is scaled by n
to maintain the average infected period even when the number of stages
changes.
|
| μ |
is
the death rate and we assume that ν=μ
|
n
|
is
the number of stages in the infected period.
|
m
|
is
the number of stages in the
exposed period.
|
| S(0) |
is
the vector of initial
proportions of the population that are both susceptible
|
| I(0) |
is
the vector of initial
proportions of the population that are both infected ( Ii(0)=I(0)/n )
|
All rates are
specified
in days.
Requirements.
All parameters must be positive, S(0) + I(0)
≤ 1,
m < n
Files
C++ Program, Python Program, Fortran Program, Parameters, MATLAB Code.
|