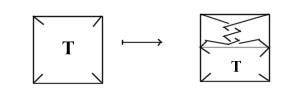
Definition   An n-twist is applied to a tangle T by choosing an adjacent pair of ends and twisting them around each other producing n crossings, where n is an integer. eg a North 3-twist:

Definition   A rational tangle is the result of applying a sequence of twists (various directions) starting with 0 or .

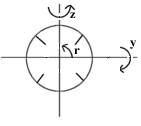From the symmetry in the definition it is clear that the dihedral group of 8 elements D₈=< r,y | ry = yr³, r⁴ = 1 = y²> acts on the set of (isotopy classes of) rational tangles, where r acts by rotation about the x-axis through 90⁰ and y is rotation through 180⁰ about the y axis. Let x = r². Let also z = xy then z acts by rotation through 180⁰ about the z-axis. The following is perhaps a little surprising.

Proposition  x, y, z operate trivially on rational tangles.

Proof  Since z = xy it will be sufficient to prove that x and z operate trivially. Clearly for one n-twist applied to 0 or the result holds. The proof now proceeds by induction on the number of twists applied.
Case i)   If the twist is east or west then y operates trivially, since we have yR = R by induction:
To see that z operates trivially operate on zR by yⁿ thus transferring crossings from west to east say and use yⁿzR = R by induction:

Case ii)   If the n-twist is north or south then z operates trivially and y can be seen to operate trivially much as in case i).

Remark  The operation on part of a knot or link given by:

We now define a basic tangle (corresponds to rational tangle in the lectures) to be a rational tangle obtained from 0 or by using only south and east twists. By using flypes and the the proposition we can convert west twists into east twists and north twists into south twists. Hence we have:

Proposition  Every rational tangle is isotopic to a basic tangle.